Multiplication Facts Strategy for Upper Grades {Freebies}

Do you have students in your 4th-6th grade class who just can’t seem to master their multiplication facts? No matter what you try (flashcards, incentives, computer based programs), they just can’t get past their 5s (and are shaky with their 3s and 4s)? I have always had several students in my classrooms over the years that really struggled memorizing and recalling their multiplication facts so I feel your pain if you are in this situation. It can be very frustrating, to say the least.

I knew I needed something other than my go-to flashcards, so I researched a few multiplication facts strategies and finally found one that I knew would help my students.

Using the Distributive Property of Multiplication to Master Multiplication Facts

I will tell you that it is not a “quick fix” strategy.

However, this is a “I have got to be able to figure out what 7 x 8 is to solve this multi-digit problem, and I don’t have time to draw a picture of 7 circles with 8 dots inside each circle.”

Yes, I literally had students in 5th grade who resorted to this each year.

And I also have had students who just sit there for several minutes because they don’t know 7 x 8 and were not taught any strategies to help them figure it out.

I knew I had to change something the year that half of my 5th grade class had not mastered their math facts.

This particular strategy that I tried (and used with success) has the students using the distributive property of multiplication, with a bit of additional scaffolding and support.

When I first introduced this strategy to my struggling multiplication students, I immediately saw light bulbs go off.  They were deepening their conceptual understanding of multiplication and feeling confident because they were able to solve the problem.

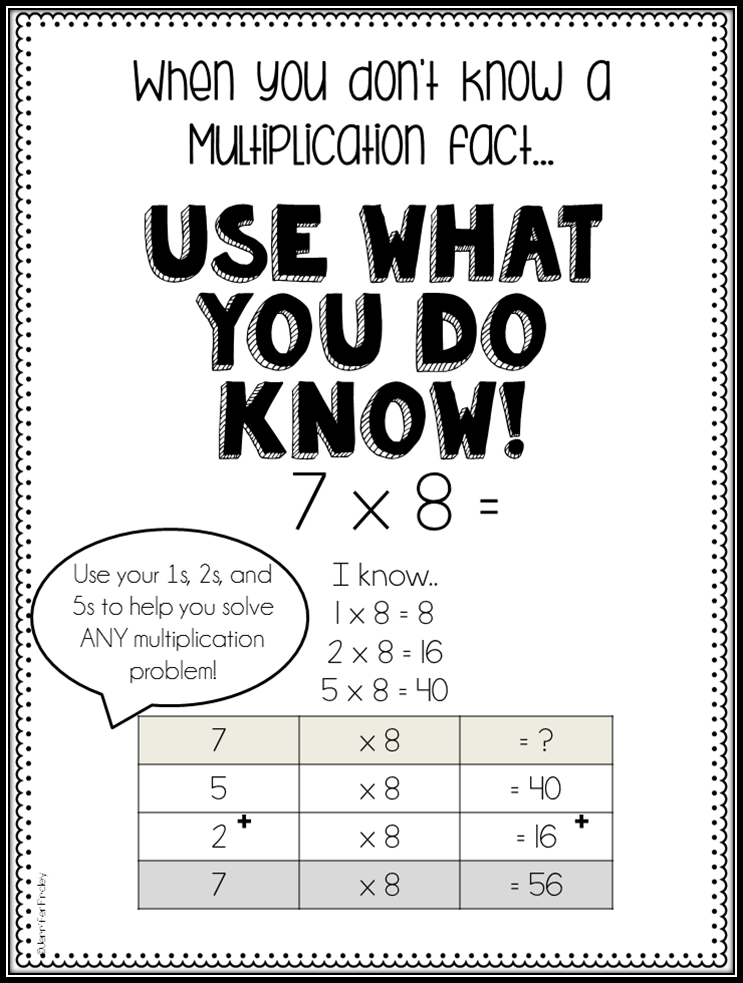
For the strategies, the students use what they know (their 1s, 2s, and 5s) to determine the answer, using the distributive property of multiplication.

Here is an example:

The student in the above example knows that 5 x 8 = 40, and 2 x 8 = 16, so the two products added together give you 56, which is the answer to 7 x 8.

Writing out their known facts (1s, 2s, 5s) provided the extra support and scaffolding that many of my students needed.

Introducing the Multiplication Strategy

When I first introduced this multiplication strategy to my students, I made sure to discuss the numbers and math in a conceptual way to ensure they understood the concept.

For example, when discussing the equation, I would say 7 groups of 8 (versus 7 times 8). Then when I refer them to their “known facts: 1s, 2s, and 5s,” I would say something like: “You need 7 groups of 8. Which of your known facts will give you the largest number of groups, without going over what you need?”

After the student selects 5 x 8 (or 5 groups of 8) we talk about how many more groups are needed to make 7 groups, etc.

Then we talk about why adding the products works. I really try to get them to conceptually understand why this strategy works.

This keeps them from making mistakes, and eventually leads to more efficient thinking and using other benchmark math facts that students with strong number sense can do. (i.e. I know that 6 x 8 = 48, so I just need another group of 8 to find 7 x 8)

Example In Action

Here is an example of one of my student’s work when he used this multiplication strategy for 9 x 6.

He marked out the 5 x 6 after he used it because he knew that he could not use it again. He then used the 2 x 6 twice.

Note: Eventually, the students will begin to make connections between the numbers and do 5 x 6 and 4 x 6, but this will take some time.

Final Thoughts and Grab a Freebie

Click here to grab your copy of the poster shown in this post as well as a few printables that I use when teaching this strategy.Save

Save

Save

Save

Save

Save

Save

Save

Save

Save