Teaching students to add and subtract fractions can get very tiresome quickly. All of the little opportunities for errors with making equivalent fractions, adding/subtracting, and just when they think they are done…boom…they may have to convert or simplify the answer. It can definitely get tedious quickly. One area that I have almost perfected (because nothing is perfect in teaching) is teaching my student to make common denominators. On this post, I will share four ways I teach students to make common denominators, the order I teach each way, and my rationale behind it.
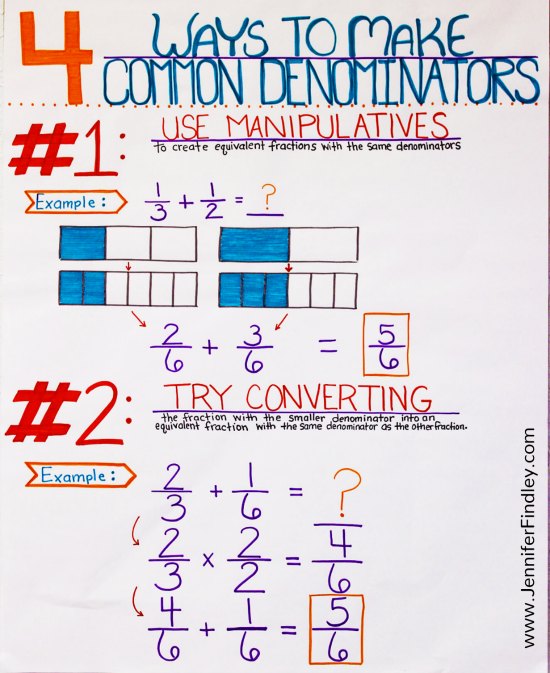
Use Manipulatives to Find Equivalent Fractions
This one is pretty much just what it sounds like. The students use manipulatives, either fraction strips or an equivalent fraction chart (similar to what is shown on the chart shown below) to convert the fractions to equivalent fractions with the same denominator.
This strategy is not something they can replicate without actual manipulatives or a similar resource, but it builds the conceptual understanding that the students are finding equivalent fractions.
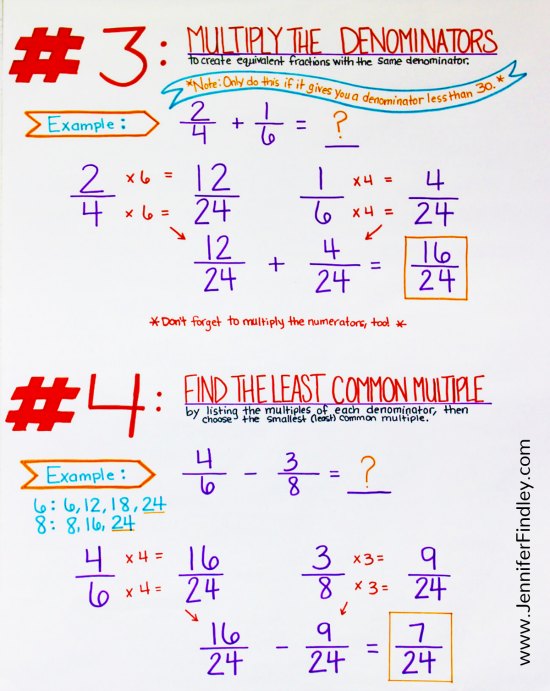
Find the Least Common Multiple
This is the trusty strategy that we all know and love. For this strategy, the students list out the multiples for each denominator and choose the least common multiple as your denominator. I have my students list out the first five because typically that is all they need to do. When I teach this strategy, I explicitly connect it to the work we did with manipulatives.
Similarly, when we talk about the next two strategies, we connect them back to the strategy of finding the least common multiple because essentially the next two strategies are “shortcuts” to finding a multiple and converting the fractions to equivalent fractions.
Convert Only One of the Fractions
As mentioned above, I teach this strategy and the next one only after I have taught least common multiple because it builds on it. However, this is one strategy that I try to get my students to use if they are able to. It saves so much time and leaves less room for error if they are only converting one fraction. It also saves them from having to list out the multiples if they can use their number sense to see if the larger denominator is a multiple of the smaller one.
For this strategy, the students examine the denominators and use what the know about multiples to determine if they can convert the fraction with the smaller denominator into an equivalent fraction with the same denominator as the other fraction. I use this language to prompt my students when working with this strategy: Is the larger denominator a multiple of the smaller denominator?
Multiply the Denominators
This is actually my least favorite strategy, but the students really love this one. I typically don’t teach this and only address it if it comes up from the students. The reason I don’t love it is that it can be seen as a trick and students can just blindly do it without really understanding what they are doing. However, it really is helpful for some students.
For this strategy, the students multiply the denominators by each other to create equivalent fractions with same denominator. I do tell them to only do this if it gives them a denominator less than 30. Anything above 30 just gets too big and complicated when they go to simplify their answers.
Anchor Chart and Free Printable
After teaching the four strategies for converting fractions to like denominators, I will bring out the anchor chart that serves as a review and also as a way to discuss a “strategy” for which strategy to use.
I have about half of the chart prepared beforehand (everything but the example work). We work on the same anchor chart for a couple of days (pacing depends on the students). We practice the strategies several times as review on markerboards before writing the example problem together on the chart and on their printables. The printables stay in their math notebooks for them to refer back to as needed.
Click or on the image below to grab a free printable that matches the anchor chart.
Common Questions
Do you teach all four ways?
Not always. It depends on my students’ needs but for the most, I do wind up teaching all four ways or addressing them at some point. I have found that a lot of my students are taught the multiply the denominator trick by older siblings. That is the only strategy that I don’t always teach but do address if it comes up.
How do you sequence introducing and teaching the different ways?
I follow this sequence when introducing and teaching the different strategies. I find this sequence builds a strong conceptual foundation and each way builds on the other for a sophisticated understanding while still having that necessary foundation.
- Using Manipulatives to Convert to Equivalent Fractions
- Finding the Least Common Multiple
- Converting Only One of the Fractions (by discussing that the larger denominator is a multiple of the smaller denominator)
- Multiplying the Denominators
However, on the chart and the printables, I list the strategies in the order that my students typically take (Omitting #3 if I choose not to use that one with that group of students).
Do you need more fraction resources for your classroom?
Click on the links below to check out a few recommended resources or articles for fractions.
4th Grade Fraction Review Mini-Booklet: I use this resource from my TeachersPayTeachers store before I teach 5th grade fraction skills. It allows me to quickly review 4th grade fraction skills and to make sure my students have the conceptual foundation needed.
Free Fraction Mats: I use these free downloadable fraction mats to support my students further as they add and subtract fractions with unlike denominators.
FREE Fraction Pacing Guide: This blog post shares an “I Can” checklist for students and shares how I pace my 5th grade fraction skills.
Chocolate Themed Fraction Math Tasks: These tasks in my TeachersPayTeachers store are perfect for engaging fraction practice with a yummy chocolate theme.
Subtracting Mixed Numbers with Regrouping Resource: This TpT resource has a pizza theme and really helps my students conceptually understanding regrouping with mixed numbers.
Subtracting Mixed Numbers with Regrouping Using Manipulatives: This blog post shares three ways you have help your students subtract mixed numbers in a more concrete way using manipulatives.
Multiplying and Dividing Fractions: When you get ready to move on from adding and subtracting fractions, this is the perfect one stop resource for multiplying and dividing with fractions.
You are AMAZING!!! Thank you for creating such an easy to follow article on this topic.
Thank you!!!! I have used several of your Freebies and TP resources. You are consistently a go to resource when Im like, ” I need a good ______” to supplement what I have already or what the curriculum lacks :/