Many of my students in the past have come to me lacking conceptual understanding of basic math concepts that were introduced as early as 2nd and 3rd grade. The most important part of my math instruction is fostering a conceptual understanding and this is really important as fifth grade moves into more abstract math concepts. With that in mind, this is the first concept lessons I teach every year to my fifth graders. To see my beginning of the year math charts that I make the first weeks of school, click here.
Note: I will also make notes of any changes I would make when I do the lesson again the next year. As teachers, we are constantly growing and improving in the same manner as we want our students and I want to make sure I share any improvements with you as well.
Having a conceptual understanding of basic multiplication and division is key in 5th grade. In 5th grade, we move into decimal and fraction multiplication which requires the students to understand that multiplication is also taking part of a whole or part of a part. However, if they don’t even have the basic understanding of multiplication, this can be very tricky for them. I keep this lesson VERY simple, but it is one of the interactive notebook entries that some students refer back to all year.
Reviewing Multiplication
To start, we talk about what multiplication is. Some students can rattle off “repeated addition” or “equal groups” but I want them to be able to give examples and discuss it in their own words. This is one key difference between a rote memorization of a concept and a deeper understanding.
After discussing that, we move into defining each number in a multiplication problem. This leads to a discussion that in multiplication of whole numbers (I do make the distinction with the students to plant the seed for decimal and fraction multiplication), you have groups and are looking for the total amount of something. This comes in handy when we move into multiplication and division word problems with larger numbers. I am constantly asking the students: What are you looking for? I want them to understand that if they have equal groups and are looking for the total amount, they are multiplying.
We also discuss arrays and rates when we talk about multiplication. I want the students to see the connection between rate problems and equal groups.
Here are some shots of the interactive template that I used. Much more discussion went into this and unfortunately a lot of that discussion was not captured in this particular student’s notes.

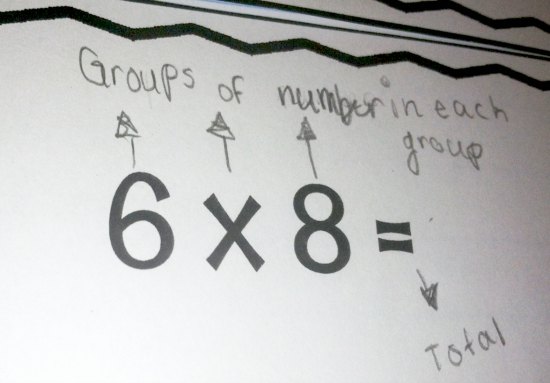
Changes to this Part of the Lesson
Here are some things I would change to improve this lesson for the next year:
- Have the students draw multiple representations of multiplication versus just the basic circle representation. Instead, we will draw arrays and number lines in addition to the circle representation for each operation.
Reviewing Division
After reviewing the concept of multiplication, we move straight into reviewing the concept of division. We follow the same steps as we did with multiplication. We discuss in depth what division is and what it means. I want the students to see the connection between multiplication and division so we use the phrase, “putting a total amount of something into equal groups.” This connects right back with the concept that multiplication is determining the total amount in equal groups. Division, on the other hand, is taking a total amount of something in putting it into groups. This is the language I use with the students. It really clicks with a lot of them that had never really grasped the connection between the two.

After discussing the basic concept of division, we move into the two types of division: number of groups unknown and group size unknown. This is really difficult for some of my students. I have to think it is because they are not exposed to those terms and/or not asked to solve a variety of division problems. With that in mind, the students complete two different models to show both types of division. Moving forward, I will definitely have the students label each model as “Group Size Unknown” or “Number of Groups Unknown.”
I refer back to these interactive math templates throughout our multiplication and division unit. This is actually the first interactive notebook entry of the year, and one of the most important. By the time we move into decimal and fraction multiplication, the students are ready to understand this aspect of multiplication.
Changes to this Part of the Lesson
Here are some things I would change to improve this lesson for the next year:
- Have the students use the term “partitioning” in addition to “putting”. This is not only more mathematical correct, but also prepares them for division of unit fractions when they are partitioning a whole into fractional amounts.
- I would use multiple representations in the same manner as I would for the multiplication part of the lesson.
Download the Multiplication and Division Templates Here
Next Steps
Here is what I do in the days following this lesson:
- Give an exit slip the next day asking the students to define multiplication and division and depending on the class, also compare the two operations.
- Pose a multiplication or division word problem a day for the students to solve. I would monitor the students closely and see which ones struggle. Grab some free word problems that are perfect for this by clicking here.
- Pull small groups for continued instruction using manipulatives for the students who need more intensive instruction on multiplication and division.
- Assess my students with their math fact fluency and send home Multiplication and Division Take Home Kits with the ones who struggle.
Do your students come to you lacking a basic conceptual understanding, or are they pretty strong in multiplication and division concepts?

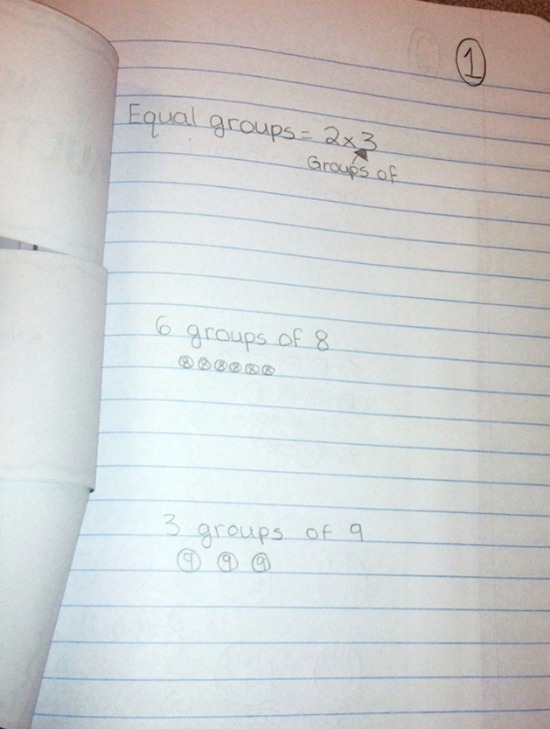






Ahh! I love this!! I’ve taught middle and high school math, and you’d be amazed at how many kids come through not understanding what multiplication and division are. It totally limits their ability to understand any of the algebra we do. Thanks so much for teaching this concept.
Yes, it is very limiting! We seriously refer back to this lesson all year for some of my students. They really need that understanding to move forward in their mathematical thinking. Thanks for stopping by!
I totally understand where you are coming from. The vertical articulation SAYS they should understand this, but they don’t. My grade level sat down with fourth grade this week to discuss with them what EXACT verbiage and ideas they need to put out in their first couple units this year to help prepare the kids for us next year and one of our suggestions was to take it alllllll the way back to place value. If the kids don’t understand that place value has a relationship of x10, they struggle with everything (double digit multiplication included)!! Thanks for these, I’m going to put them to use when I do my review next week!
Brianna
Yes, place value and just basic number sense is also something many of my students struggle with each year. I always *try* to fix the roadblocks early on before we get into the more abstract math. Thanks for stopping by!
As a 4th grade teacher, I’m excited about using these ideas with my students. Hopefully I can lay a good foundation for them as they use these concepts in later years.
Hi could u expand on the division of the groups size unknown and number of groups unknown. I have never heard of this before. Thanks
Hi Alex, here are examples that may clear it up:
Number of Groups Unknown: Jackson has fifty-six pieces of candy. He wants to make treat bags with 4 pieces of candy in each. How many bags will he be able to make? In this problem, we know how many goes in each group, but the number of groups is unknown.
Size of Groups Unknown: Jackson has fifty-six pieces of candy. He wants to divide the candy equally among 4 bags. How much candy will be in each bag? In this problem, we known how many groups, but the size of the groups is unknown.
Hope this helps! Thanks for visiting!
Jennifer
With interest have i read your post. I like your emphasis of teaching “groups” in multiplication it appears to me that this needs to stressed much further than one might think. I came across this article today as i was searching for an understanding of why people have a lack of understanding for the rule that multiplication comes before addition 3+3×5=18. Interestingly in the course of the conversation it become apparent that people follow “order of operations” in the english language . I am a non-native english speaker. During the course of the conversation it was reasoned that the order of operations is followed because someone said so. It was followed up with reasoning that math would work as well if one was to define addition comes before multiplication 4+3×5=35. This apparent relativism leds me to believe that there is a lack of understanding of what addition and multiplication means. Applying this way of thinking in maths would require a new math to adhere to the basics of counting. Rather then teaching a rule, children need to understand that addition can be of the same kind and also of different kinds while a multiplication means of one kind exclusively. A multiplication as you all know is a addition of the same number for a defined amount. Once a child is aware that there is a set theory, i believe the “order of operations” is self explanatory and does not need to be memorized by a sentence. In a very simple way 3 apples +3 times 5 pears will be 18 fruit or 3 apples and 15 pears. The way how an equation is read is defined from left to right and also linguistically multiplication is defined from left to right. 2 times 5 even though that 2 times 5 could be expressed as 5+5 or 2+2+2+2+2 to illustrate mathematics it is counterproductive as 2 times 5 is 5+5 otherwise it is 5 times 2. To conclude by understanding of what multiplication is it is very unlikely one would conclude 3 apples+3 times 5 pears could be 3 apples and times multiplied by 5 pears. I believe once this is understood by children that advanced math is easier and the understanding of why multiplication comes before addition comes automatically as well as the point of why there are no parentheses needed for a multiplication. I think that becomes illustrative when children have to do inventories. Set scene is a total of 30 items. This is the sum. The sum breaks up in 10 bottles and 1 jam and 1 jam and 1 jam and 17 candies. To express this in a shorter way multiplication was developed. 30= 10 bottles+3x1jam+17 candies. It seems very simple but this simple math can be formulated into advanced formular that applies to set scene: 30=10xa+3xc+17xb resulting in 3 unknowns with a rule,that indicates the total number of items won’t change neither the amount of each category will change but the items themself can be different.
I love the way you inspire me. I am going to have this handy way for my youngest daughter. She will be a 4th grader next July. Someone used to tell me about the conceptual understanding as the first decisive stage, out of five consecutive stages of doing with mathematics. And you gave me this wonderful concrete example.
Would you, please, bring me to a further trip of working with children on this. Once the children get the concept of multiplication and division through “equal groups”, how do you take them into the prosedural fluency? I imagine that you use sticks, blocks or whatever stuff to help children putting total into groups. If so, how do children move this concrete things into their abstract mental operation? How long does it usually take for them to be independent from the concrete procedures?
Thank you.
Best Regards,
Yanto
I am interested in this question as well! It is tricky making that bridge.
thank you